Pacific Coast Am at Olympic

[Tom Huckaby]

anybody know why this tournament is conducted at stroke play?

Only guessing here - but since a big part of it is the Morse Cup (team competition) - and that would be damn impossible to do as match play - stroke play it is.

[Kevin_Reilly]

Unless they actually build a tee for the 3rd hole ON the chipping green, all the tees that can be built, have been built!

They could tear down the clubhouse, create more length for number one.  

It's the pro shop that would need moving....it would be easier to move the green...plenty of room for that.  Not that I'm in favor of it, since I'm not.

[Mike Benham]

anybody know why this tournament is conducted at stroke play?

With 18 Pacific Rim golf associations comprising the Pacific Coast Golf Association, how would you organize match play without the risk of eliminating half the field on Day 1 (which would dissuade the Associations from spending the money to send teams to the event)?

From the PCA website:

The Morse Cup Team competition has been a major portion of the PCGA Championship since 1967.  Each team consists of three players. From 1967 to 1996, the best two scores each day for the first two rounds were totaled for each team to determine the Morse Cup Champion. In the event of a tie, co-champions were recognized. However, the team play ended after 36 holes.

A significant change in the Championship format occurred in 1997. This change made it possible for golf associations throughout the international golf scene to become a part of this storied event. When the 31st annual competition began on July 29, 1997 , at Makena Resorts South Course in Maui , Hawaii , the emphasis was on team play and scores were counted from all four rounds. This format change opened the door to include teams of three players representing other national and regional associations, as well as member associations of the PCGA.  

In 2002, Trustees of the PCGA voted to return to the tournaments original format, a moved spurred in large part by the fact that the USGA does not recognize results from team competitions in making selections for Walker Cup squads.  The Pacific Coast Amateur is now an individual competition with all competitors playing 72 holes without a cut being made. The Morse Cup competition continues to be held in conjunction with the Championship, but only PCGA member associations are allowed to have teams.[/i]

[Tom Huckaby]

Mike - I could have saved you some time - see two posts above.

 

[Tiger_Bernhardt]

There are some great events in the world of Am golf.

[Mike Benham]

Mike - I could have saved you some time - see two posts above.

 

You were guessing, I was confirming ...

[Kevin_Reilly]

Here is the field...with teams and individual invitees.  Buddy Marucci among the latter.

Field for Pacific Coast Am

Course stats from play on the Ocean Cse.

Hole by Hole Stats

[Mike Benham]

Here is the field...with teams and individual invitees.  Buddy Marucci among the latter.

Field for Pacific Coast Am

LIVE SCORING at the Pacific Coast Amateur

Ps:  They are playing the Ocean course today ...

[Kevin_Reilly]

Field averaged 74.56 (par 71) on the Ocean...interesting that #18 played as the 6th most difficult hole...usually plays much easier for everyday play.

[Joel_Stewart]

Field averaged 74.56 (par 71) on the Ocean...interesting that #18 played as the 6th most difficult hole...usually plays much easier for everyday play.

Really surprising it was that high but I haven't played there in a month so I don't know the conditions.  With the heat last week the greens could have been really fast.  As for #18, it depends on the pin position because I can't imagine thos guys not having wedges into that hole???

How does Buddy Marucci shoot that high on the Ocean course?

[Mike Benham]

Field averaged 74.56 (par 71) on the Ocean...interesting that #18 played as the 6th most difficult hole...usually plays much easier for everyday play.

That is surprising ... I checked the weather cam and the wind mph and direction was normal.  Perhaps many of the young guys tried to drive it to the bottom of the hill only to find trouble ... or a tight hole location ...

[David_Tepper]

Buddy Marucci's 79 was not the only high score to raise a few eye brows. OC member (and several time club champ) Randy Haag shot 76. SF City champ Will Johnson shot 77. Cal State Am champ Jordan Nasser shot 78.    

Golf is a tough game and the Ocean is no easy course!!!

[AndrewB]

So the work on the Ocean course's 15th green a 16th tee is done then, I take it.  Can anyone comment on how significant the changes turned out to be and how they affect play of the holes?

[Matt_Cohn]

So, I will be at Olympic tomorrow morning. Not sure what time but...hopefully by 8-something or 9:00 at the latest. I'm taking BART and walking but if anyone wants to offer me a ride at the last minute, please message me!

Hope to see some of you there.

[JohnV]

I was a trustee for the Pacific Coast Am in 1997 when we voted to emphasize the team format.  The reason was that by being a team competition the associations could pay the expenses of the players.

Given the new rules on expenses the issue is moot now.

The purpose of the PCA was always to show off the West Coast players for the USGA Walker Cup selections.  By getting Buddy Marucci out there this year they are doing a good job of that.  I'm surprised that it is the same week as the Western Am this year as we usually tried to avoid that conflict as it definitely hurt the field quality.

[David_Tepper]

Thru two rounds, NO ONE is under par!

[Kevin_Reilly]

Lake played one stroke more difficult on average than the Ocean...stroke average was  75.5 today.  

Most interesting, to me, was the relative difficulty of #5...it ranked as the fourth most difficult hole today with an average of 4.489.  I would have guessed that with the tree clearing on the right side that it would have played easier than that.  

Maybe Matt can give us a report on today's play when he gets home later.

[Mike Benham]

Kevin -

I was just looking at the stats ... pretty tough stretch of golf from 2 though 5 ...

For this player of golfer, I don't think the clearing of the trees on the right would make the hole that much easier, primarily because I don't expect them to miss right as the average golfer.  And if they are hitting 3-wood off the tee, the aren't trying to cut the corner or play along the tree line ...

How much harder will #2 play when the new tees go into play?

Hole   Yds  Par   Average   Rank
2   394   4   4.456        5   
3   229   3   3.567        3   
4   438   4   4.578        2   
5   457   4   4.489        4

#17 was the hardest hole, being played as a 464 yard par 4 at a stroke average of 4.633.

Mike

[Matt_Cohn]

I'm happy to give a report, especially because I have a couple of excellent stories to go with it.

The golf course was set up similarly to how I saw it for members' play except that the greens were about a foot faster. The pins were challenging but not extreme by any means - mostly 6-8 yards from the edges. The rough didn't seem deeper than normal. There was some swirling wind and I think that affected scores a bit. The difference from the members' setup was 2 shots at most.

The fairways at Olympic are in the best condition of almost any course I've ever seen. The greens are good for poa annua but everyone had a couple of putts bounce at some point during the round. The talk I heard was that the greens on the Ocean were not quite as good, and that this affected scoring.

Regarding #5, the wind and pin position were tough. The wind was to the left off the tee and swirling in the fairway. The pin was 21 on and 6 from the right - probably the toughest area of that green. Most of the guys hit driver off the tee which probably isn't the correct play with that wind. A case of bad club selection more than bad swings. Balls were in the left rough, not the right trees.

OK, on to my stories. These are good ones!

1. I got to Olympic just before my friend Henry Liaw teed off. (He started on #9.) I actually haven't seen Henry in a long time. He's had a great college career at Arizona and has a bunch of European Tour exemptionsl lined up for when he turns pro later this summer.

I said hello, and I heard him mention to a playing partner that he hadn't played a practice round on the Lake. (Also in his pairing was Clay Ogden, the past Public Links champion forever known as the guy who beat Michelle Wie.) I bit my tongue as he predictably misjudged several shots.

As we walked off #14 tee, I mentioned that I wished I could guide him around, or at least point out things like, "Hey, that's the 6th green over there." He said it would help and I told him I'd check with a rules official first. The rules official said that I couldn't say anything unless I was officially his caddy, and according to this official, I couldn't just call myself his caddy - I had to actually carry the bag, rake bunkers, etc.

I politely asked him if this was written anywhere in the rule book. He drove off and came back about five minutes later. His answer was, as long as I wore a caddie bib, I could say anything I wanted. I told him that worked for me, and five minutes later - we were on #16 green by now - he came back with a bib for me. I asked Henry if he really wanted to go through with this and he said unhesitatingly that he really needed the help getting around the course! I was officially Henry's caddy!

The first thing he said was that I didn't have to carry the bag. The first thing I said was, "Dude, I'm not gonna be the only guy in a caddy bib who's not carrying anything. I'm carrying your bag!"

So off we went. He was +3 when I picked up his bag on #17 tee; he played -1 the rest of the way in to finish with a respectable 72. I gave him some good advice - the highlight was when I changed his read on a 35 footer on #2 that he proceeded to hole for a nice birdie. I had a lot more fun caddying than just watching, and I like to think that I helped him shoot a lower score too.

2. Buddy Marrucci, the great amateur player, played in the group ahead of ours and didn't have his best day. I had to go to work at Golfsmith immediately after the round, and I was quite shocked at around 6:00 when who walks into Golfsmith but...Buddy Marrucci!? So that was a surprise. I enjoyed walkin into the office and telling my managers, "Umm, the captain of the Walker Cup team is in the hitting bay on the right...."

By the way, Buddy is an exceptionally nice guy. I really enjoyed spending about 20 minutes talking to him and showing him a some items in the store.

But even more surprising was when I realized he was actually there to buy a set of irons, which he eventually did. So now I can't help but hope for him to play well the next two days!

Anyway, that was my day at Olympic. I'll be back on Friday, I think - maybe on Henry's bag, maybe not. Again, message me if you'll be around.

Cheers,
Matt

[James Bennett]

EDIT - HELP - THE DAMN TABLE AND GRAPH BELOW ARE TOO SMALL TO READ.  ANY SUGGESTIONS WITH PHOTOBUCKET?  THEY ARE EXCEL CHARTS.
END OF EDIT, MY APOLOGIES FOR THE SMALL TABLE/CHART


Matt

great to hear you had a good day - your efforts to get there deserved it.

This thread has encouraged me to develop an analysis I have thought about before but hadn't done before.  It uses the data from the Ocean course players (90 rounds) and compares the average score of each hole, the standard deviation on each hole (measuring the spread of scores) and presenting the outcome graphically.

The graph has the holes in length order, par 3's first and par 5's last (I saw this on Mike Nuzzo's work, and more recently on Stanley Thompson's via Geo Thomas's book Golf Architecture in America).  The hole numbers are on the x-axis, the yardage of the hole on the left hand axis and the average score on the right axis.  The average score is shown as a colored square, the lower value of the blue line for a hole is average less one standard deviation, the upper value is average plus one standard deviation.

I have chosen this to try to statistically show which holes provide the greatest variety of outcomes (I think TEPaul has a name for this, but I can't remember it).  So, the standard deviation statistic can distinguish between two holes which average say a par of 4  over 90 rounds - one hole has 80 pars, 5 birdies and 5 bogies whilst the other hole has 20 birdies, 51 pars 18 bogies and a double bogey.  Both may average 4 but the level of interest and opportunity to test skills is quite different.

I have updated the data from the Pacific Coast Amateur by adding the std deviations in the following table.  The chart in yardage order follows.





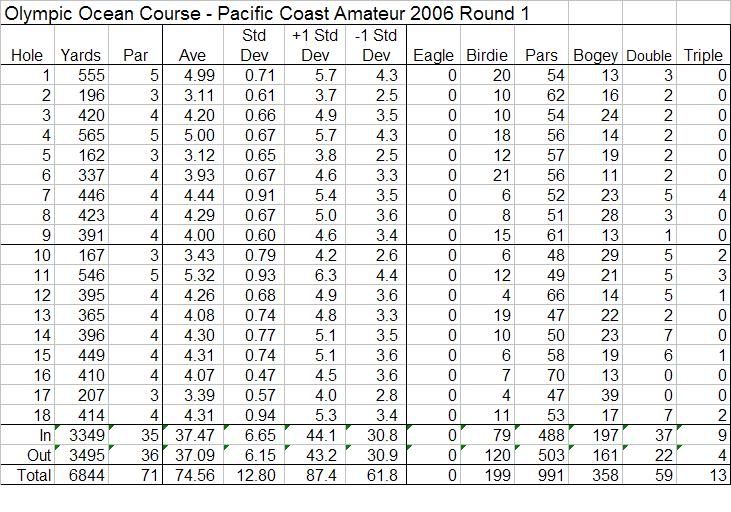
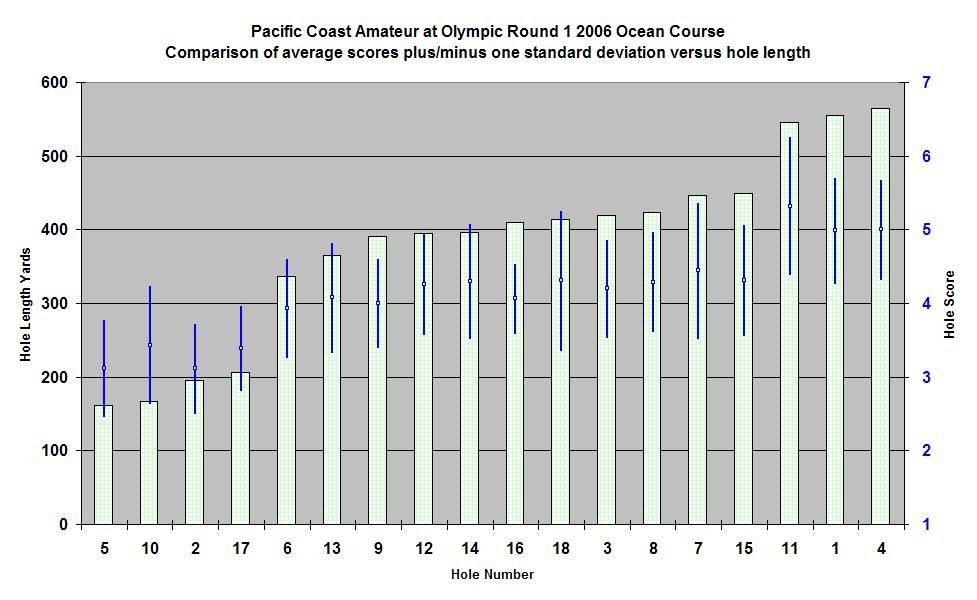
For an initial analysis, consider the 4 par 3's.  Look at the average scores, and the spread of scoring.  The two shorter par 3's have played more difficult and with a wider spread.  I haven't seen the holes, but I expect the shot required on these holes is more exacting but one which can obtain a birdie reward if well executed.

Then consider the 3 par 5's.  The 11th is slighly shorter but plays on average more difficult than the others, with a massive scoring spread.  There must be a variety of hazards that come into play on this hole.

Also consider the 16th hole.  Very little scoring spread on this hole - it has the highest frequency of par scores across the days play.  The 17th hole also has a small scoring range (std deviation).  However, the 18th hole has the widest spread (std deviation) of all holes.

You may have some other comments on the charts.  Has this analysis been done before?  Is it worthwhile?  Perhaps it implies a method for determining a matchplay stroke index (greatest variation is hole number 1) if a reasonable sample is used.  Perhaps the analysis can be used to help determine and test some alternative course set-up issues.  Perhaps its just rubbish or has already been done before.

I might add the Lake course results to the thread later on the weekend.  I would also be interested to look at say the 4 days of the Master's as 4 separate analyses (if I can find the data), to see how much hole location/setup/conditions will effect the std deviation.  I'd also be interested to see how the std deviations fall out on the back nine.  It is the most exciting TV golf, I wonder what the stats say.

James B

[David_Tepper]

Matt -

Sounds like you had quite a day. Two questions:

1) Were you surprised that a player of Henry Liaw's caliber would not have played a practice on a course with the pedigree of the Lake Course in a tournament as prestigous as this one?

2) Did you talk with Buddy Marucci at all about what he thought of the OC courses? I am curious to know what his opinions might be.

DT  

[Tom Huckaby]

David - I don't presume to answer for Matt - but re:

1) Were you surprised that a player of Henry Liaw's caliber would not have played a practice on a course with the pedigree of the Lake Course in a tournament as prestigous as this one?

I have to ask:  when was he supposed to get in such practice round?  The club was generous to offer such prior to the tournament, but space was very limited (from what I read).  And if he's not from the area and/or has no way to access the club, well...

I guess I wouldn't be surprised at all if many of the players in this event played the Lake course sans practice round.  But I do look at things differently.

TH

[Kevin_Reilly]

For an initial analysis, consider the 4 par 3's.  Look at the average scores, and the spread of scoring.  The two shorter par 3's have played more difficult and with a wider spread.  I haven't seen the holes, but I expect the shot required on these holes is more exacting but one which can obtain a birdie reward if well executed.

It will be interesting to see how #5 plays today..there are several tee boxes on this hole - an extreme drop-shot short hole.  These fellows shouldn't have trouble with it, but if there is any wind to speak of, it could make the hole more difficult.  #2 and #17 are rather straightforward.  #10 is exposed to wind and has many hazards around the green.

Then consider the 3 par 5's.  The 11th is slighly shorter but plays on average more difficult than the others, with a massive scoring spread.  There must be a variety of hazards that come into play on this hole.

All on the tee shot, which must carry a barranca.  The second shot (assuming someone is going for the green) is uphill and semi-blind.

Also consider the 16th hole.  Very little scoring spread on this hole - it has the highest frequency of par scores across the days play.

Straightforward hole - no hazards.

The 17th hole also has a small scoring range (std deviation).

Straightforward par 3.

 However, the 18th hole has the widest spread (std deviation) of all holes.

I'm not sure why this is the case...normally a fairly routine hole.  maybe the rough lines are a factor for the tournament?

Thanks for the charts James...hope all is well in Oz.  Hope the Wallabies get back on track versus SA.

[Matt_Cohn]

Henry had just gotten to California from the Porter Cup. His lack of a practice round was simply due to his schedule, not any access issues. I'm not sure how much of the field was able to play two practice rounds.

Buddy said he liked the Lake Course very much and thought the Ocean Course was fine except that the greens weren't rolling that well. (I never played the Porter Cup but it sounds like the Porter Cup course isn't really that great. The guys were happy to be at a place like Olympic!) Everyone said that the Lake was 2-3 shots harder relative to par which is about what the results showed.

[Tom Huckaby]

Matt - I don't know Henry Liaw from Adam Ant, so have no clue about his access issues.  I guess I was just assuming at least SOME of the competitors in this event were regular joes, and thus would have "access issues"... not to mention the fact quite a few might be coming to the area just for this event, and with practice rounds limited, well...

Oh well... no biggie... David's question just to me seemed strange.  

 

[Tags:]

Tags: